Sectional forces

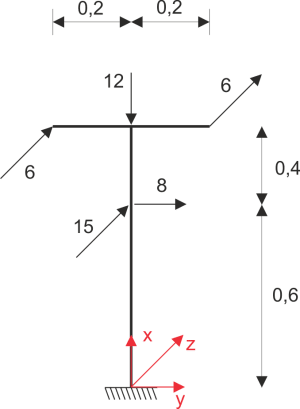\begin{aligned} &Q_{z}=15 k N \\ &Q_{y}=8 k N \\ &Q_{x}=N=-12 k N \\ &M_{z}=0 \\ &M_{y}=-15 \cdot 0.6=-9 k N m \\ &M_{x}=-6 \cdot 0.2-6 \cdot 0.2=-2.4 k N m \end{aligned}

Tangential stresses - shearing

Stresses caused by force \(Q_z\)

\begin{aligned} &\tau_{x z}=\frac{4}{3} \cdot \frac{Q_{z}}{A}\left(1-\frac{z^{2}}{R^{2}}\right) \\ &A=\frac{\pi \cdot 0.2^{2}}{4}=0.03 m^{2} \\ &\tau_{x z}^{A}=\frac{4}{3} \cdot \frac{15 \cdot 10^{3}}{0.03}\left(1-\frac{0.2^{2}}{0.2^{2}}\right)=0 \\ &\tau_{x y}=-\frac{4}{3} \cdot \frac{Q_{z}}{A} \frac{y z}{R^{2}} \\ &\tau_{x y}^{A}=0 \end{aligned}

Stresses caused by force \(Q_y\)

\begin{aligned} \tau_{x y} &=\frac{4}{3} \cdot \frac{Q_{y}}{A}\left(1-\frac{y^{2}}{R^{2}}\right) \\ \tau_{x y}^{A} &=\frac{4}{3} \cdot \frac{8 \cdot 10^{3}}{0.03}\left(1-\frac{0}{0.2^{2}}\right)=0.356 \mathrm{MPa} \\ \tau_{x z} &=-\frac{4}{3} \cdot \frac{Q_{y}}{A} \frac{y z}{R^{2}} \\ \tau_{x z}^{A} &=0 \end{aligned}

Tangential stresses - torsion

\begin{aligned} &\tau_{x y}=-\frac{M_{x}}{I_{o}} z \\ &I_{o}=\frac{\pi d^{4}}{32}=1.57 \cdot 10^{-4} \mathrm{~m}^{4} \\ &\tau_{x y}^{A}=-\frac{-2.4 \cdot 10^{3}}{1.57 \cdot 10^{-4}} \cdot 0.1=1.53 M P a \\ &\tau_{x z}=\frac{M_{x}}{I_{o}} y \\ &\tau_{x z}^{A}=0 \end{aligned}

Total tangential stresses

\begin{aligned} &\tau_{x y}^{A}=1.53+0.356=1.886 \mathrm{MPa} \\ &\tau_{x z}^{A}=0 \\ &\tau^{A}=\sqrt{1.886^{2}+0^{2}}=1.886 \mathrm{MPa} \end{aligned}

Normal stresses

\begin{aligned} &\sigma=\frac{N}{A}+\frac{M_{y}}{I_{y}} z-\frac{M_{z}}{I_{z}} y \\ &I_{z}=I_{y}=\frac{\pi d^{4}}{64}=7.85 \cdot 10^{-5} \\ &\sigma^{A}=\frac{-12 \cdot 10^{3}}{0.03}+\frac{-9 \cdot 10^{3}}{7.85 \cdot 10^{-5}} \cdot 0.1=-11.86 \mathrm{MPa} \end{aligned}

Reduced stresses

\begin{aligned} &\sigma_{\text {red }}^{H M H}=\sqrt{\sigma^{2}+3 \tau^{2}} \\ &\sigma_{\text {red }}^{H M H} A=\sqrt{(-11.86)^{2}+3 \cdot 1.886^{2}}=12.3 M P a \end{aligned}