Solution
1. Marking characteristic points and reactions on supports
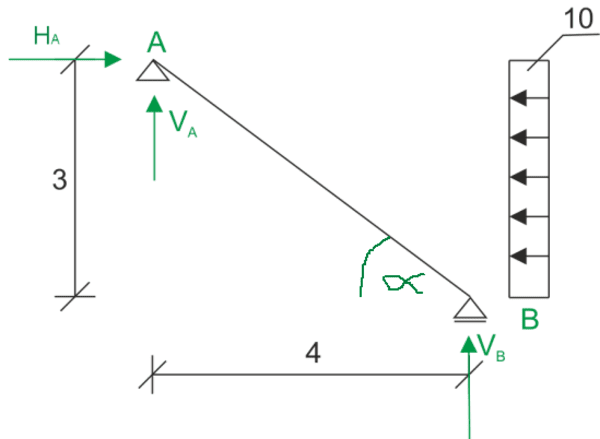 \begin{aligned} &\sum{M_A}=0\\ &-V_B\cdot 4+10\cdot 3\cdot 1,5=0 &V_{B}=11.25\\ &\sum{F_Y}=0\\ &V_{A}=-11.25\\ &\sum{M_B}=0\\ &-V_{A}\cdot 4+H_{A}\cdot 3-10\cdot 3\cdot 1.5=0 &H_{A}=30\\ \\ \end{aligned}
\begin{aligned} &sin\alpha=0.6\\ &cos\alpha=0.8\\ \\ \end{aligned}
\begin{aligned} &\sum{M_A}=0\\ &-V_B\cdot 4+10\cdot 3\cdot 1,5=0 &V_{B}=11.25\\ &\sum{F_Y}=0\\ &V_{A}=-11.25\\ &\sum{M_B}=0\\ &-V_{A}\cdot 4+H_{A}\cdot 3-10\cdot 3\cdot 1.5=0 &H_{A}=30\\ \\ \end{aligned}
\begin{aligned} &sin\alpha=0.6\\ &cos\alpha=0.8\\ \\ \end{aligned}
We decompose reactions into components along and perpendicular to the axis of the rod:

Section AB
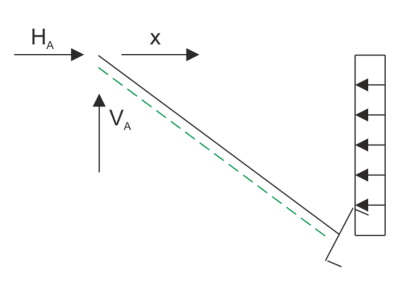 \begin{aligned} &N_{AB}=-H_{A}\cdot cos\alpha+V_{A}\cdot sin\alpha+10\cdot cos\alpha\cdot y\\ &N_{A(0)}=-30.75\\ &N_{B(2)}=-6.75\\ &Q_{AB}=H_{A}\cdot sin\alpha+V_{A}\cdot cos\alpha-10\cdot sin\alpha\cdot y\\ &Q_{A(0)}=9\\ &Q_{B(4)}=-9\\ \\ &M_{AB}=H_{A}\cdot y+V_{A}\cdot x-10\cdot \frac{y^2}{2}\\ &M_{A(0)}=0\\ &M_{B(4)}=0\\ &M_{MAX(2)}=11.25 \\ \end{aligned}
\begin{aligned} &N_{AB}=-H_{A}\cdot cos\alpha+V_{A}\cdot sin\alpha+10\cdot cos\alpha\cdot y\\ &N_{A(0)}=-30.75\\ &N_{B(2)}=-6.75\\ &Q_{AB}=H_{A}\cdot sin\alpha+V_{A}\cdot cos\alpha-10\cdot sin\alpha\cdot y\\ &Q_{A(0)}=9\\ &Q_{B(4)}=-9\\ \\ &M_{AB}=H_{A}\cdot y+V_{A}\cdot x-10\cdot \frac{y^2}{2}\\ &M_{A(0)}=0\\ &M_{B(4)}=0\\ &M_{MAX(2)}=11.25 \\ \end{aligned}
Internal force diagrams

If you have any questions, comments, or think you have found a mistake in this solution, please send us a message at kontakt@edupanda.pl.
