Solution
TASK PROCEDURE - method of forces
SSN=4-3=1
Basic System of the Method of Forces (BSMF)

State \(X_1=1\)
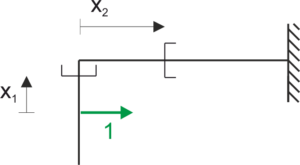
Bending moment function
\begin{aligned} &x_{1} \in(0 ; l) \\ &M_{1}\left(x_{1}\right)=-1 \cdot x_{1} \\ &x_{2} \in(0 ; 4 l) \\ &M_{1}\left(x_{2}\right)=-l \end{aligned}State P
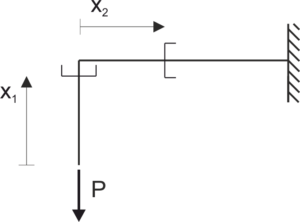
Bending moment function
\begin{aligned} &x_{1} \in(0 ; 2 l) \\ &M_{p}\left(x_{1}\right)=0 \\ &x_{2} \in(0 ; 4 l) \\ &M_{p}\left(x_{2}\right)=-P \cdot x_{2} \end{aligned}Canonical equation of the Method of Forces
\begin{gathered} \delta_{11} \cdot x_{1}+\delta_{1 p}=0 \\ E I \delta_{11}=\int_{0}^{L}\left(M_{1} \cdot M_{1}\right) d x=\int_{0}^{l} x^{2} d x+\int_{0}^{4 l} l^{2} d x=\frac{1}{3} l^{3}+4 l^{3}=\frac{13}{3} l^{3} \\ \delta_{11}=\frac{13}{3 E I} l^{3} \\ E I \delta_{1 p}=\int_{0}^{L}\left(M_{1} \cdot M_{p}\right) d x=\int_{0}^{4 l} P l x d x=P l \cdot \frac{1}{2} \cdot(4 l)^{2}=8 P l^{3} \\ \delta_{1 p}=\frac{8 P l^{3}}{E I} \\ x_{1}=\frac{-8 P l^{3}}{\frac{13}{3} l^{3}}=\frac{-24}{13} P \end{gathered}After calculating the statically indeterminate reactions, we calculate and draw the final diagrams.
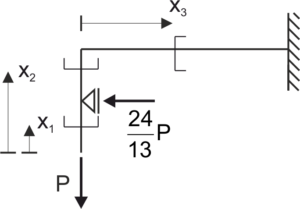 \begin{aligned}
&x_{1} \in(0 ; l) \\
&N\left(x_{1}\right)=P \\
&T\left(x_{1}\right)=0 \\
&M\left(x_{1}\right)=0 \\
&x_{2} \in(l ; 2 l) \\
&N\left(x_{2}\right)=P \\
&T\left(x_{2}\right)=\frac{24}{13} P \\
&M\left(x_{2}\right)=\frac{24}{13} P\left(x_{2}-l\right) \\
&M(l)=0 \\
&x_{3} \in(0 ; 4 l) \\
&N\left(x_{3}\right)=\frac{24}{13} P \\
&T\left(x_{3}\right)=-P \\
&M\left(x_{3}\right)=-\frac{28}{13} P l+P x \\
&M(0)=-\frac{28}{13} P l\\
&\quad M(2 l)=\frac{24}{13} P l
\end{aligned}
\begin{aligned}
&x_{1} \in(0 ; l) \\
&N\left(x_{1}\right)=P \\
&T\left(x_{1}\right)=0 \\
&M\left(x_{1}\right)=0 \\
&x_{2} \in(l ; 2 l) \\
&N\left(x_{2}\right)=P \\
&T\left(x_{2}\right)=\frac{24}{13} P \\
&M\left(x_{2}\right)=\frac{24}{13} P\left(x_{2}-l\right) \\
&M(l)=0 \\
&x_{3} \in(0 ; 4 l) \\
&N\left(x_{3}\right)=\frac{24}{13} P \\
&T\left(x_{3}\right)=-P \\
&M\left(x_{3}\right)=-\frac{28}{13} P l+P x \\
&M(0)=-\frac{28}{13} P l\\
&\quad M(2 l)=\frac{24}{13} P l
\end{aligned}
Final internal forces diagrams

If you have any questions, comments, or think you have found a mistake in this solution, please send us a message at kontakt@edupanda.pl.
