Edupanda » Strength of materials » Wereszczagin Method
Wereszczagin Method
↓ link to a database of tasks with solutions and video courses ↓
See 3 simpler examples from this section!
-> within our subscription for access
to all content on our website <-
See 7 more difficult examples from this section!
-> within our subscription for access
to all content on our website <-
See 7 examples in Gerber beams!
-> within our subscription for access
to all content on our website <-
See 9 examples in FRAMES!
See a free video course from this section!
See the second free video course from this section!
Introduction to the Wereszczagin Method
step-by-step presentation
To practice, we leave you with the calculation of the rotation angle at point A, expect a result of (=-12.5/EI) :-)
So Wereszczagin noticed that
\(u=\int \frac{M_g \cdot M_1}{E I} \cdot d x=\frac{1}{E I} \cdot \Omega \cdot \eta\)If we need to integrate together figures (meaning functions), where:
- one is or can be parabolic (quadratic function, under constant continuous load) - it will be the diagram in the Initial State (from given loads),
- the other is non-parabolic - it will be the Unit State, when calculating displacements this will be the diagram from the unit force applied at the point and in the direction of the desired displacement - See more on this topic - Maxwell-Mohr Method,
then the result of such integration can be reduced to the formula:
\(u=\Omega \cdot \eta\)
where:
\( \Omega \) - area of the figure on the moment diagram in the Initial State,
\( \eta \) - ordinate on the diagram in the Unit State at the position (at the height) of the center of gravity of the figure from the Initial State.
The following image shows this graphically.

A few practical notes
1) What does it actually mean if the displacement is negative?This means that the direction of the displacement will be opposite to the direction of the assumed unit force.
In the case of vertical displacement at point B, a unit force with downward direction was assumed,
the displacement came out negative, which means that in reality it will be upward.
The direction of the unit force corresponding to the desired rotation angle at point A was assumed to be clockwise.
2) When integrating using the Wereszczagin method, we need to pay attention to whether the areas of the integrated figures are on the same side of the beam or on the opposite side.
If they are on the same side, the result of this single integration is positive, if they are on opposite sides, it is negative.
3) Another important thing is the division into simple figures. Both the diagram in the Initial State and in the Unit State, if they are more complicated than: rectangle, right triangle, parabola, we can and will mentally divide them into simple figures.
Examples of division into simple figures
Trapezoid 1
An example similar to the one we considered when deriving the Wereszczagin method.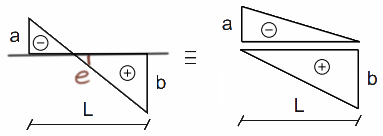
Trapezoid 2
Now a figure that we actually identify at first glance as a trapezoid.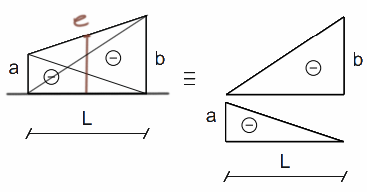
Sometimes it is useful to calculate the value at the midpoint of the diagram, how can this be done easily?
If the diagram changes linearly, then at the midpoint there is the arithmetic mean of the two extreme values, for example:
- on the first plot \( e=\frac{-a+b}{2} \),
- on the second plot \(e=\frac{-a-b}{2} \),
If the diagram changes parabolically, then to this mean value we need to add or subtract the value \( f=\frac{q\cdot l^2}{8} \) representing the bulge of the parabola that occurs if we have a continuous load on the element.
So \( e=\frac{a+b}{2}\pm \frac{q\cdot l^2}{8} \), where whether we give plus or minus depends on whether the action of the continuous load causes the parabola to be convex or concave, but see the examples below.
Triangle-parabola
The action of the continuous load causes the parabola to be convex, so \( e=\frac{0+a}{2}+\frac{q\cdot l^2}{8} \)
Parabolic trapezoid 1
Now something more interesting and in fact nothing more difficult than two further cases can happen.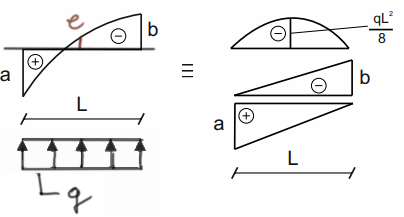
\( e=\frac{a+(-b)}{2}-\frac{q\cdot l^2}{8} \)
Parabolic trapezoid 2
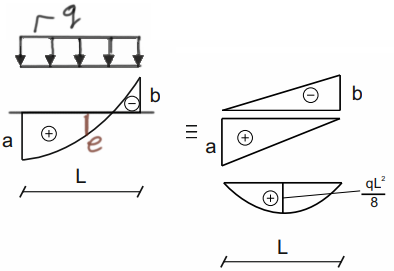
\( e=\frac{a+(-b)}{2}+\frac{q\cdot l^2}{8} \)
Parabolic trapezoid 3
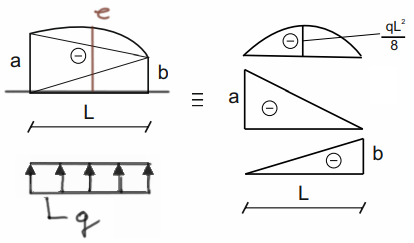
\( e=\frac{-a+(-b)}{2}-\frac{q\cdot l^2}{8} \)
Parabolic trapezoid 4
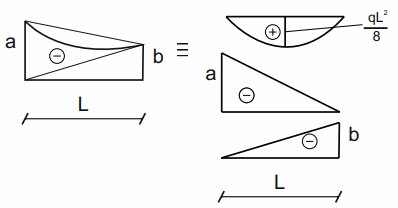
\( e=\frac{-a+(-b)}{2}+\frac{q\cdot l^2}{8} \)
Parabolic trapezoid 5
I think the principle of operation is already clear.Finally, one more, troublesome at the beginning case. We must remember that if the continuous load is not perpendicular to the rod, then when distributing into simple figures, when calculating the height of the parabola \( f \) as the length, we take the length of the continuous load, and not the length of the rod!.
See the example below
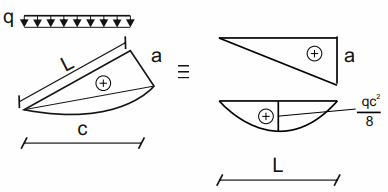
\( e=\frac{0+a}{2}+\frac{q\cdot c^2}{8} \)
Wereszczagin formulas/table
Derived formulas are often also used using the Wereszczagin method.Basically, all possible integrations can be calculated using four formulas:
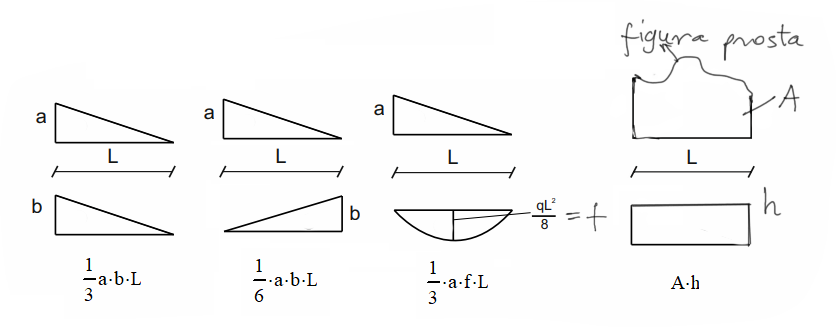
With each integration, we pay attention to the signs of the integrated figures, if they are the same, we have a positive result, if they are opposite, we have a negative result.
Formulas:
1) Triangle with a triangle -> if the heights are on the same side, according to the formula for \( \Omega \cdot \eta = \frac{1}{2}\cdot a \cdot L\cdot \frac{2}{3} \cdot b \) which gives us after shortening the ready formula \( \frac{1}{3} \cdot a \cdot b \cdot L \)
2) Triangle with a triangle, heights on opposite sides -> \( \Omega \cdot \eta = \frac{1}{2}\cdot a \cdot L\cdot \frac{1}{3} \cdot b=\frac{1}{6} \cdot a \cdot b \cdot L \)
3) Triangle (arbitrarily rotated) with a parabola -> Attention! When integrating with a parabola, the area must be calculated from the parabola, and the ordinate from the second figure. \( \Omega \cdot \eta = \frac{2}{3}\cdot f \cdot L\cdot \frac{1}{2} \cdot a=\frac{1}{3} \cdot a \cdot F \cdot L \)
4) Any figure with a rectangle -> according to the definition, it will be the area of this arbitrary figure times the ordinate of the rectangle, which has the same height "h" everywhere. \( \Omega \cdot \eta = A \cdot h \)
Where any figure, after decomposition into simple figures, will be:
- triangle
- rectangle
- or parabola
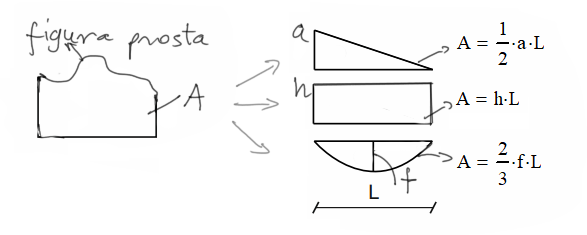
Some people use more formulas, you can multiply them really many, but these four plus the decomposition into simple figures are enough to deal with any situation.
Of course, calculating from the definition and not remembering ready-made formulas will always be fine.
(AGH, Podstawy Mechaniki Budowli, Kraków 2003)
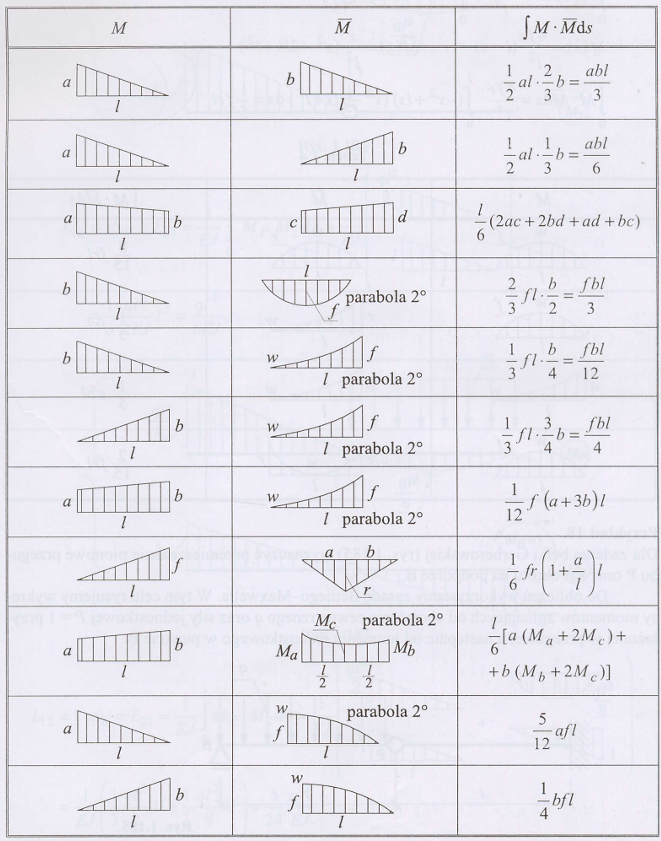
The third formula from the top and the third from the bottom are particularly popular among AGH students :)
Examples of calculating displacements in beams using the Wereszczagin integration method
↓ link to the database of tasks with solutions and to video courses ↓
See 3 simpler examples from this section!
-> within our subscription for access
to all content on our website <-
See 7 more difficult examples from this section!
-> within our subscription for access
to all content on our website <-
See 7 examples in Gerber beams!
-> within our subscription for access
to all content on our website <-
See 9 examples in FRAMES!
Watch free video course from this section!
Watch second free video course from this section!
See the offer and price of tutoring

|
Łukasz CichowiczTel: +48 780 155 029E-mail: lukasz@edupanda.pl Skype: edupandapl |